Study Guide: Functions and Control

Instructions

This is a study guide with links to past lectures, assignments, and handouts, as well as additional practice problems to assist you in learning the concepts.

Draw environment diagrams automatically with Python Tutor.

Assignments

Important: For solutions to these assignments once they have been released, see the main website

Lab 1
HW 1

Handouts

Discussion 1

Lectures

Readings

Guides

`return` vs. `print`

A lot of students were confused about the return statement (more specifically return vs. print).

return statements allow the programmer to return a value from a function. You can take the returned value and save it to a variable or do whatever you want with it.

Think of it as a store. When you return something at the store, that item that you return will probably be purchased by someone else and they will use it however they want. (I know the analogy is not so great).

print statements on the other hand just print what you want to the screen and returns None. It doesn't allow you to actually use the value that you printed elsewhere. Once a value is printed, Python can no longer use it for computation.

Two different functions will be used to illustrate the difference.

The first function example will return a string:

def some_function():
    return 'I love John DeNero'

Calling some_function returns the string, 'I love John DeNero', so I can also bind that return value to a name.

my_love = some_function()

Now, the variable my_love is set to the return value of some_function(), in this case it is 'I love John DeNero'. So my_love = 'I love John DeNero'.

The second function example will print a string:

def some_function():
    print('I love John DeNero')

Calling some_function() now will print 'I love John DeNero' on the terminal and then return None. (Recall that, if a function has no return statement, it will return None.) This time, binding the return value to a name has a different result.

my_love = some_function()

It will print 'I love John DeNero' on the terminal and bind my_love = None.

It is important to note that return statements will terminate the function.

def some_function():
    return 5
    return 3

It will return 5 and end the function without ever returning 3.

Boolean context

Boolean contexts are "places in Python code where you place an expression but all that matters about that expression is whether it's true or false."

Here's an example from lecture of a function containing an if statement with two boolean contexts.

def absolute_value(x):
    """Return the absolute value of x."""
    if x < 0:
        return -x
    elif x == 0:
        return 0
    else:
        return x

In the if clause, Python asks, "Is x < 0 a true value or a false value?" This depends on the value of x. Suppose we have x = 3.

>>> x = 3
>>> x < 0
False

Python evaluates the call expression x < 0 to False. Now, we need to ask the question, is False a false value? This might seem like an unusual question to ask, but it's actually a very subtle step.

In Python, false values include the following values (more to come):

False, 0, '', None

Everything else is a true value.

Because we know that False is a false value, the entire expression x < 0 is a false value in boolean context.

Let's look at another example to see why boolean context and true value/false value distinction matters. Suppose we want to evaluate the following boolean expression.

>>> print('hi') or 25

The or operator contains two boolean contexts, one on each side of the or. Let's evaluate this expression.

print('hi') prints the string 'hi' to the terminal and returns None. Because None is a false value in Python, the first boolean context is false.

25 evaluates to the number 25. Because 25 is a non-zero number, it is a true value in Python and so the second boolean context is true.

The final value that is returned from the entire or is 25 because Python uses the boolean context only to determine how to interpret the value in the context of an or expression. 25 is not the same as True; it just evaluates to a true value in boolean context.

WWPD Questions

For each of the expressions below, write the output displayed by the interactive Python interpreter when the expression is evaluated. The output may have multiple lines. If an error occurs, write "Error", but include all output displayed before the error. If a function value is displayed, write "Function".

Recall: The interactive interpreter displays the value of a successfully evaluated expression, unless it is None.

Assume that you have started python3 and executed the following statements:

Q1: Multiples

from math import sqrt

x = 15

def square(x):
    return print(x * x)

def multiply(x):
    x_new = x * 2
    return x * 3

>>> print(print(3, 5))
______
3 5
None
>>> square(x)
______
225
>>> True and 17
______
17

>>> print(multiply(x))
______
45
>>> multiply(multiply(3))
______
27
>>> print(square(multiply(2)), 8) + 3
______
36
None 8
Error

Q2: Quizzical

x = 2

def take_quiz(x):
    print(x * 10)
    if x * 10 >= 100:
        return 'Good job!'
    return 'Go to office hours!'

def office_hour(x):
    while x < 10:
        x = x + 3
    return print(take_quiz(x))

>>> pow(print(0, 0), 0)
______
0 0
Error
>>> office_hour(4)
______
100
Good job!

>>> print(take_quiz(x))
______
20
Go to office hours!
>>> print(office_hour(x))
______
110
Good job!
None

>>> office_hour(office_hour(x))
______
110
Good job!
Error

>>> take_quiz(-3) and print(office_hour(x))
______
-30
110
Good Job!
None

Q3: There can only be Wan

Hint: Draw environment diagrams to track progress! Don't just quietly recite the names to yourself, as names that sound similar can easily be mixed up!

def wan1(wan1, wan11):
    return wan1 == wan11

def wan11(wan11, wan1):
    return wan1 / wan11

one, wan, won = 1, 3, 17

def derek(wan, won, one):
    print(wan1(wan, wan11(won, 1)))
    return wan11(one, wan1)

>>> print(one or wan11(0, one))
______
1
>>> print(wan1(one, wan11(one, one)))
______
True

>>> print(print(wan - won), wan1)
______
-14
None Function

>>> derek(1, 1, 2)
______
True
Error

>>> derek(wan, 2, one)
______
False
Error

Q4: Whoos hat?

s = 2

def hat(s):
    while s > 0:
        whoos(s)
        s = s - 1
    return whoos(s)

def someones(their, hat):
    his, her = their, hat
    hat = print(his or her) and her or his
    if hat:
        return 'hat'

def whoos(hat):
    return print(hat)

>>> print(s) or 1 / 0
______
2
Error
>>> 0 or 2 == True and print(5)
______
False

>>> her, cat = 'her', print('theirs') or 'his'
______
theirs
>>> someones(her, cat)
______
her
'hat'

>>> hat(s)
______
2
1
0

>>> print(whoos(hat), someones(cat, hat))
______
Function
his
None hat

Q5: Out at the Ballgame

def announce(score, inning):
    hits = score * 3 % inning - 1
    print('The Giants have ' + str(score) + ' runs!')
    if hits < 2:
        jumbotron_text = print('That\'s the end of the inning!')
        inning = inning + 1
        return jumbotron_text
    else:
        score = score + hits % 3
        crowd_noise = print('The Giants now have: ' + str(score))
        print(str(crowd_noise))
    return score

def player(hits, inning):
    if hits < 2:
        print('I need to practice in the batting cage!')
    score = hits * 2 + 1
    saying = announce(score, inning)
    return saying * 2

>>> print(3, print(5 % 3))
______
2
3 None

>>> player(0, 0)
______
I need to practice in the batting cage!
Error

>>> player(3, 2)
______
The Giants have 7 runs!
That's the end of the inning!
Error

>>> player(5, 9) - 5
______
The Giants have 11 runs!
The Giants now have 13
None
21

>>> player(announce(15, 8), 4)
______
The Giants have 15 runs!
The Giants have 16
None
The Giants have 33 runs!
The Giants now have 35
None
70

Practice Problems

Easy

Q6: Distance

Implement a function called distance(x1, y1, x2, y2):

x1 and y1 form an x-y coordinate pair
x2 and y2 form an x-y coordinate pair

distance returns the Euclidean distance between the two points. Use the following formula:

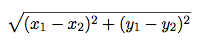
2-D distance formula

from math import sqrt

def distance(x1, y1, x2, y2):
    """Calculates the Euclidian distance between two points (x1, y1) and (x2, y2)

    >>> distance(1, 1, 1, 2)
    1.0
    >>> distance(1, 3, 1, 1)
    2.0
    >>> distance(1, 2, 3, 4)
    2.8284271247461903
    """
    "*** YOUR CODE HERE ***"
    return sqrt(square(x1-x2) + square(y1-y2))

Q7: Distance (3D)

Now, let us edit this program to get the distance between two 3-dimensional coordinates. Your distance3d function should take six arguments and compute the following:

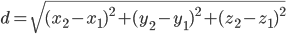
3-D distance formula

def distance3d(x1, y1, z1, x2, y2, z2):
    """Calculates the 3D Euclidian distance between two points (x1, y1, z1) and
    (x2, y2, z2).

    >>> distance3d(1, 1, 1, 1, 2, 1)
    1.0
    >>> distance3d(2, 3, 5, 5, 8, 3)
    6.164414002968976
    """
    "*** YOUR CODE HERE ***"
    return sqrt(square(x1-x2) + square(y1-y2) + square(z1-z2))

Q8: Harmony

Implement harmonic, which returns the harmonic mean of two positive numbers x and y. The harmonic mean of 2 numbers is 2 divided by the sum of the reciprocals of the numbers. (The reciprocal of x is 1/x.)

def harmonic(x, y):
    """Return the harmonic mean of x and y.

    >>> harmonic(2, 6)
    3.0
    >>> harmonic(1, 1)
    1.0
    >>> harmonic(2.5, 7.5)
    3.75


    >>> harmonic(4, 12)
    6.0
    """
    "*** YOUR CODE HERE ***"
    return 2/(1/x + 1/y)

Q9: Environments

Python Tutor is a great visualization tool for environment diagrams. Paste in your Python code and it will generate an environment diagram you can walk through step-by-step! Use it to help you check your answers!

Try drawing environment diagrams for the following examples and predicting what Python will output:

>>> def square(x):
...     return x * x
>>> def double(x):
...     return x + x
>>> a = square(double(4))
>>> a
______
64

>>> x, y = 4, 3
>>> def reassign(arg1, arg2):
...     x = arg1
...     y = arg2
>>> reassign(5, 6)
>>> x
______
4
>>> y
______
3

>>> def f(x):
...     f(x)
>>> print, f = f, print
>>> a = f(4)
______
4
>>> a
______
# Nothing shows up, because a = None
>>> b = print(4)
______
4
>>> b
______
# Nothing shows up, because b = None

Q10: Fix the Bug

The following snippet of code doesn't work! Figure out what is wrong and fix the bugs.

def compare(a, b):
    """ Compares if a and b are equal.

    >>> compare(4, 2)
    'not equal'
    >>> compare(4, 4)
    'equal'
    """
    if a = b:
        return 'equal'
    return 'not equal'

The line a = b will cause a SyntaxError. Instead, it should be

if a == b:

Q11: Last square

Implement the function last_square, which takes as input a positive integer and returns the largest perfect square less than its argument. A perfect square is any integer multiplied by itself:

Hint: If you're stuck, try writing a function that prints out the first 5 perfect squares using a while statement: 1, 4, 9, 16, 25. Then, adapt that while statement to this question by changing the header.

def last_square(x):
    """Return the largest perfect square less than X, where X>0.

    >>> last_square(10)
    9
    >>> last_square(39)
    36
    >>> last_square(100)
    81
    >>> result = last_square(2) # Return, don't print
    >>> result
    1


    >>> cases = [(1, 0), (2, 1), (3, 1), (4, 1), (5, 4), (6, 4),
    ...          (10, 9), (17, 16), (26, 25), (36, 25), (46, 36)]
    >>> [last_square(s) == t for s, t in cases].count(False)
    0

    """
    "*** YOUR CODE HERE ***"
    k = 0
    while k * k < x:
        k = k + 1
    return (k-1) * (k-1)

We iterate over perfect squares until we find the first one larger or equal to the input. The answer is then the square before that one. This solution is inefficient, but an efficient solution requires taking a square root.

Q12: Overlaps

An open interval is a range of numbers that does not include its end points. For example, (10, 15) stands for all numbers that are strictly greater than 10 and strictly less than 15. Two intervals overlap if they contain any points in common. For example (10, 15) overlaps (14, 16), but not (1, 5) or (15, 16). The intervals (10, 10) or (10, 9) contain no numbers, since nothing is both greater than and less than 10, or greater than 10 and less than 9. Implement the function overlaps to take four numbers as arguments, representing the bounds of two intervals, and return True if the intervals overlap and False otherwise.

def overlaps(low0, high0, low1, high1):
    """Return whether the open intervals (LOW0, HIGH0) and (LOW1, HIGH1)
    overlap.

    >>> overlaps(10, 15, 14, 16)
    True
    >>> overlaps(10, 15, 1, 5)
    False
    >>> overlaps(10, 10, 9, 11)
    False
    >>> result = overlaps(1, 5, 0, 3)  # Return, don't print
    >>> result
    True


    >>> [overlaps(a0, b0, a1, b1) for a0, b0, a1, b1 in
    ...       ( (1, 4, 2, 3), (1, 4, 0, 2), (1, 4, 3, 5), (0.1, 0.4, 0.2, 0.3),
    ...         (2, 3, 1, 4), (0, 2, 1, 4), (3, 5, 1, 4) )].count(False)
    0
    >>> [overlaps(a0, b0, a1, b1) for a0, b0, a1, b1 in
    ...       ( (1, 4, -1, 0), (1, 4, 5, 6), (1, 4, 4, 5), (1, 4, 0, 1),
    ...         (-1, 0, 1, 4), (5, 6, 1, 4), (4, 5, 1, 4), (0, 1, 1, 4),
    ...         (5, 5, 3, 6), (5, 3, 4, 6), (5, 5, 5, 5),
    ...         (3, 6, 5, 5), (4, 6, 5, 3), (0.3, 0.6, 0.5, 0.5) )].count(True)
    0
    """
    "*** YOUR CODE HERE ***"
    return low1 < min(high0, high1) > low0

There are many solutions to this problem. One way to look at it is to consider conditions under which the intervals don't overlap. Clearly for two non-empty not to overlap, one has to come entirely before the other. This becomes high1 <= low0 or high0 <= low1, which when negated is high1 > low0 and high1 > low1. In addition, both lower bounds must be less than their respective upper bounds (or the intervals are empty). The solution given combines these observations.

Q13: Triangular numbers

The nth triangular number is defined as the sum of all integers from 1 to n, i.e.

1 + 2 + ... + n

The closed-form formula for the nth triangular number is

(n + 1) * n / 2

Define triangular_sum, which takes an integer n and returns the sum of the first n triangular numbers, while printing each of the triangular numbers between 1 and the nth triangular number.

def triangular_sum(n):
    """
    >>> t_sum = triangular_sum(5)
    1
    3
    6
    10
    15
    >>> t_sum
    35
    """
    "*** YOUR CODE HERE ***"
    count = 1
    t_sum = 0
    while count <= n:
        t_number = count * (count + 1) // 2
        print(t_number)
        t_sum += t_number
        count += 1
    return t_sum

Medium

Q14: Same hailstone

Implement same_hailstone, which returns whether positive integer arguments a and b are part of the same hailstone sequence. A hailstone sequence is defined in Homework 1 as the following:

Pick a positive integer n as the start.
If n is even, divide it by 2.
If n is odd, multiply it by 3 and add 1.
Continue this process until n is 1.

def same_hailstone(a, b):
    """Return whether a and b are both members of the same hailstone
    sequence.

    >>> same_hailstone(10, 16) # 10, 5, 16, 8, 4, 2, 1
    True
    >>> same_hailstone(16, 10) # order doesn't matter
    True
    >>> result = same_hailstone(3, 19) # return, don't print
    >>> result
    False


    Extra tests:

    >>> same_hailstone(19, 3)
    False
    >>> same_hailstone(4858, 61)
    True
    >>> same_hailstone(7, 6)
    False
    """
    "*** YOUR CODE HERE ***"
    return in_hailstone(a, b) or in_hailstone(b, a)

def in_hailstone(a, b):
    """Return whether b is in hailstone sequence of a."""
    while a > 1:
        if a == b:
            return True
        elif a % 2 == 0:
            a = a // 2
        else:
            a = a * 3 + 1
    return False

Q15: Nearest Power of Two

Implement the function nearest_two, which takes as input a positive number x and returns the power of two (..., 1/8, 1/4, 1/2, 1, 2, 4, 8, ...) that is nearest to x. If x is exactly between two powers of two, return the larger.

You may change the starter implementation if you wish.

def nearest_two(x):
    """Return the power of two that is nearest to x.

    >>> nearest_two(8)    # 2 * 2 * 2 is 8
    8.0
    >>> nearest_two(11.5) # 11.5 is closer to 8 than 16
    8.0
    >>> nearest_two(14)   # 14 is closer to 16 than 8
    16.0
    >>> nearest_two(2015)
    2048.0
    >>> nearest_two(.1)
    0.125
    >>> nearest_two(0.75) # Tie between 1/2 and 1
    1.0
    >>> nearest_two(1.5)  # Tie between 1 and 2
    2.0


    >>> nearest_two(3)
    4.0
    >>> nearest_two(.01)
    0.0078125
    """
    power_of_two = 1.0
    "*** YOUR CODE HERE ***"
    if x < 1:
        factor = 0.5
    else:
        factor = 2
    while abs(power_of_two * factor - x) < abs(power_of_two - x):
        power_of_two = power_of_two * factor
    if abs(power_of_two * 2 - x) == abs(power_of_two - x):
        power_of_two = power_of_two * 2
    return power_of_two

This implementation repeatedly doubles or halves the number power_of_two until reaching the closest number to x. The last three lines enforce the tie-breaking policy when x is exactly betweeen two powers of two.

Q16: Pi Fraction

Complete the implementation of pi_fraction, which takes a positive number gap and prints the fraction that is no more than gap away from pi and has the smallest possible positive integer denominator. See the doctests for the format of the printed output.

Hint: If you want to find the nearest integer to a number, use the built-in round function. It's possible to solve this problem without using round.